29:52 Homework Set #2
Assigned:†
February 13, 2004
Due: February 20, 2004
Confused? Donít go around miserable and despondent! Ask for help and explanation from me. Even better, work on these problems with one or two of your classmates.
- Two asteroids (little planets; weíll talk about them later in the semester) are in orbit around the Sun. One has a period of 3 years, the other 10 years.† Assume the eccentricity is small (close to zero) for both orbits. Draw an accurate sketch of the two orbits.† Be sure to describe the calculations you use in making the sketch.
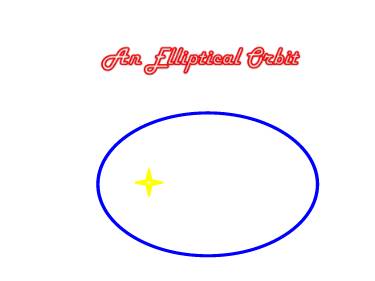
- An orbit is shown in the figure below.† What is the eccentricity of the orbit?†
- A radioisotope A decays to daughter isotope B (via Beta-decay, for example) with a half life of 1.5 billion years. You obtain a sample of a rock from the asteroid Vesta.† You analyse the rock and find that it has 10 atoms per cubic centimeter of† isotope A, †and 70 per cubic centimeter of †B.† You may assume that when the rock formed, it contained no atoms of isotope B, †in other words, all of B in the rock were produced by radioactive decay from isotope A.† How long ago did the rock form?† Show your calculations and explain what you are doing.†
- Here is one to give you an idea of the significance of astronomical timescales.† Consider the rock above.† You decide (for extra credit) to watch a movie of the surface of Vesta since the time the aforementioned rock formed.† It obviously would take a long time to watch† the movie (you anticipate some sort of toxic poisoning from† consuming an astronomical amount of Milk Duds), so you speed up the film.† You speed it up to the point where the time from the beginning of the American Revolution to the present (225 years) flashes by in five minutes.† How long are you sitting there watching the movie of the history of Vesta?
- Hereís a neat one!† A satellite is in orbit around the Earth.† The orbit has the following characteristics.† The plane of the orbit is the equatorial plane of the Earth (i.e. the Earthís equator lies in the plane).† The orbit is highly eccentric (letís say an eccentricity of 0.90).† The orbital period is 12 days.† You observe the location and motion of the satellite during the course of its orbit.† At †perigee (the point in its orbit when it is closest to the Earth) you observe it in the direction of the constellation Orion ( Right Ascension 5h30m, Declination 0degrees).† Describe where you subsequently see the satellite (i.e. in what constellations), and the characteristics of its motion against the background starfield.† This is not a quantitative problem, meaning that you do not have to carry out calculations, but you should utilize some of the scientific principles discussed in class in describing what you would observe.†
- The semi-major axis of the orbit of Mars is 1.523 astronomical units, and the eccentricity is 0.093.† What is the closest Mars comes to the Sun (the so-called perihelion distance).† What is the furthest Mars is from the Sun (the aphelion distance).† Hint:† Think of how the eccentricity is defined (problem 2 should help in this regard.† Then think of what the semi-major axis means.† Finally, where is the Sun located in the orbit?†
- This is a continuation on problem 6.† Get similar orbital data on the Earth from Appendix 5 of the book.† Calculate the closest Mars and Earth ever get to each other (this occurs at opposition).† What is the greatest distance between the two planets at opposition?†
- You look up in the sky in daytime.† You see the Moon, about 45 degrees away from the Sun.† What phase is the Moon, i.e. new,† full, half, between half and full, etc. ?